An Efficient Combination of the Denominations of a Currency
Takashi Shimazaki
Index
(1) Easiness to count amounts(2) Denominations per place
(3) Tests in coins' places
(4) Tests in banknotes' low places
(5) Tests in banknotes' high places
(6) The best C2D
(7) 1,3C's possibilities
(8) C3Ds at a glance
(9) C2D or C3D
(10) Conclusions
Abbreviations
(1) Easiness to count amounts
Money in the shape of coin or note is, nowadays, increasingly taken over by electronic money. However, as long as there remains real money in human society, it should be as much usable for everyone as possible. For example, denominations, or coins and banknotes, should be moderate in size and shape to be easily handled. It is also important that the denominations represent due values for easier calculation: if face values were oddly set, it would be laborious for people to count the amounts of money, taking more time that is a mere waste. Then, what values should denominations be?
(1.1) Head number
In the beginning, I define a word HN (Head Number) as the number without consecutive 0s from the one's place of a whole number. For example, the HN of 300 is 3, that of 81 is 81, and that of 1050 is 105. Besides, the numbers of digits of these HNs are 1, 2, and 3 respectively.
The volume of calculation depends largely on the number of digits of a HN. For instance, calculating "400+70" is easier than "388+75" despite their similar quantities. "1300-800" or "70*30" can also be counted more briefly in the human brain than "1308-761" or "74*26". Division and other arithmetic operations are omitted here because they are seldom used in counting the amounts of money. Except for the number zero, the smallest number of digits of HNs are one: such numbers are 1, 2, ... 9, 10, 20, ... 90, 100, 200, ... Then, denominations should be set at these values for easier counting.
There are currencies that have D[25] (denominations of HN=25). For example, Russian 25-ruble coin, United States 25-cent coin (quarter) are among them. If these D[25] were the minimum unit of pricing, they would make sense. But that is not the case, and so these face values are odd, only complicating cash counting. Therefore, D[25] is without reason.
(1.2) Indispensable D[1]
By the way, suppose you make an amount in cash, say Y732 (the amount of 732 yen). You would feel the amount as "700+30+2", and make 7 in 100-yen's place, 3 in 10-yen's place, and 2 in 1-yen's place. Thus, when you make an amount, you usually make the amount of each place separately. In practice, there can be situations to make every amount of money. So it is necessary to be able to make any number (1, 2, ... 9) in any place (one's, ten's, hundred's, ...), by using only the denominations of that place. To fulfill this condition, I set D[1] in each place. This is in parallel with what all the currencies do, and there seems to be no other solutions.
(2) Denominations per place
In the European Union, where the currency is the euro, the present denominations are 0.01 (1-cent), 0.02, 0.05, 0.1, 0.2, 0.5, 1, 2, 5, 10, 20, 50, 100, 200, and 500 (15 kinds in total). While in Japan, where the currency is the yen, those are 1, 5, 10, 50, 100, 500, 1,000, 5,000, and 10,000 (9 kinds in total except for a barely prevalent 2,000-yen). You can see a difference in the number of denominations between these two currencies: the euro is C3D (Combination of 3 Denominations per place), while the yen is C2D.
A C3D currency is better than a C2D one in terms of the smaller number of coins and banknotes necessary in shopping or other cash transactions. People can also carry fewer coins and notes in their wallets. At the same time, however, C3D has a wider variety of denominations than C2D, which is obstructive in differentiating them in people's wallets or in shops' cash registers.
I develop arguments about C2D before C3D. As D[1] is indispensable, the next to do is to determine the best D[N] (N=2, 3, ... 9) in each place. And these D[N]s, along with D[1]s, sum up to the best set of denominations of a currency.
Incidentally, most of the present currencies in the world have D[5], and some have D[2]. The number 5 and 2 are the divisors of 10. But this has no meaning in using cash: you count coins and notes themselves and not on your 5 fingers; when you exchange a D[1], there is no need to exchange it for a single denomination. Before further reading this paper, you need be free from such bias, which has ever prevented human beings to perceive what this paper aims to do.
(3) Tests in coins' places
To examine the efficiency of denominational combinations in detail, I investigate coins and banknotes independently. By the way, all the circulating currencies on the planet are in the form of coin or banknote. With no exception, as far as I know, coins are applied to lower places of amounts and banknotes are to higher places. This rule seems to have good reasons: giving lower values to smaller things and higher values to larger things; making cheap things hardy to endure a possible rough treat, whereas using vulnerable material for precious things that would be greeted with politeness. I have no objection to this point.
Regarding coins' efficiency, there are 2 indicators depending on the situations: one is QC (Quantity (mass or volume) of Coins) a payer carries, and the other is LC (Load of Count in cash transactions). The smaller QC or LC, the better: if QC is smaller, the wallets people carry get lighter and more compact; and if LC is smaller, people can treat cash more briefly in paying.
All the payments in cash transactions are divided into two types: TPO (Transaction of Payment Only, with change not allowed) or TPC (Transaction of Payment with Change allowed). Note that to pay without change in a transaction that allows change is classified as TPC. The proportion of TPO would be fairly small compared to that of TPC because change is allowed in most cases, otherwise retailers, including vending machines, might lose their potential shoppers.
(3.1) Test A: QC of TPO in CP
QC depends not only on NCC (the Number of Coins a payer Carries) but on the coins' mass and volume as well. Coins' mass and volume varies among currencies and among denominations, though they all seem to be suitable in size for the earth people to handle. From this point of view, it is not a good idea to make all the coins very small to lessen QC or to save minting cost. This paper does not deal with the issue of what size is ideal for coins.
What is at issue is R[N:1] (the quantity Ratio of D[N] to D[1] in the same place). To calculate QCs, I have to determine R[N:1]. So, I searched some world coins for their mass and volume. See measurements of coins. As R[N:1]s have a quite wide range, from as low as below 1 to as high as 5, I took geometric mean instead of arithmetic mean. R[N:1] of mass averaged out at 1.70 and that of volume was 1.53. The geometric mean of these two ratios is 1.61 (table 1-15). Then, QC is defined as NCC[1] (NCC of 1-yen coins) plus 1.61*NCC[N]. The ratio 1.61 applies to all CP (Coins' Places) because there would be no need to distinguish R[N:1] between coins' lower places and higher places.
To look into QC of TPO, I arrange test A with the yen. Here, test A deals with 1-yen's place only. Suppose there are enough 1-yen and N-yen coins. N is one of 2, 3, ... 9, and so there are 8 competitors, or 8 kinds of C2Ds. On test A, I first count minimum NCCs in making Y1, Y2, ... Y9 without change. Then, I calculate QC, the sum of NCC[1] and 1.61 times NCC[N]. I dare take these two steps to get QC data, imitating the real payers' reckoning: most people would think of NCC, not QC with R[N:1] in mind. This process of calculation does not make a difference here, but later it does on some tests.
Table 2-1 shows test A of 1,5C (1,5 Combination, or the combination of D[1] and D[5]), in which 1-yen coins and 5-yen coins are employed. For example, QC to make Y7 is 2+1.61=3.61. And the total QC to make Y1 to Y9 is 28.05. Thus, I investigated the other C2Ds for their total QCs as well. The results are at table 2-2.
(3.2) Test B: LC of TPO in CP
Next, I examine LCs of the 8 C2Ds. This time, the mass or the volume of coins does not matter. LC is mainly related to NCM (the Number of Coins Moved in cash transactions), that includes coins of both pay and change. But there are other factors to be considered: easiness to count amounts, which makes a difference between D[1] and D[N]. Take the hundred-yen coins, 1 coin equals Y100, 2 coins equal Y200, 3 coins are Y300, ... Thus, the HN of a sum is always equal to that of NCC[1]. On the contrary, for D[N]s, HN of a sum is never equal to that of NCC[N]. This is the reason for the HN=1's easier counting.
Taking this into account, I count HN=N (N=2~9) coins 1.5 times the actual number in calculating LC. Although easiness to count might vary among D[N]s, the gaps would be quite small compared to that between D[1] and D[N]. So, I set the constant 1.5 as common to all D[N]s. Then, LC is the sum of NCM[1] (NCM of 1-yen coins) and 1.5 times NCM[N]. I counted the total of minimum LCs to make Y1 to Y9 without change, which is test B. The results of test B are shown at table 2-3 and table 2-4.
(3.3) Test C: QC of TPC in CP
For TPC, I conducted a test of simulated transactions with the help of a computer. Test C examines the 8 C2Ds for how small their average QCs can be. And test D does how small the average LCs can be. This time, the amounts to make are randomly chosen from Y1 to Y9 in 1-yen's place as the real cash transactions. Here, I omitted Y0 that appears in reality, because it has no effect on 1-yen's place. I created a series of 100,000 random numbers of 1 to 9 to use in the simulation, and conducted cash transactions consecutively 100,000 times. The distribution of numbers 1 to 9 is at table 2-5.
On test C and test D, NCC[1] and NCC[N] of every transaction are passed on to the next transaction as they are. P (a Payer in cash transactions) possesses enough 10-yen coins in addition to some 1-yens and N-yens. 10-yens represent all the denominations of Y10 and larger in a live payer's wallet. But 10-yen coins are not counted in QC or LC as that is the matter of 10-yen's place.
On test C, which studies a minimum QC, P tries every time to best reduce the NCC. For example, table 2-6 explains how the simulated transactions of 1,6C proceed. Suppose P carries sometime no 6-yen coin, two 1-yen coins, and enough 10-yens. Now, the first amount to make is Y3, and P "pays a 10-yen coin with receiving a 6-yen and a 1-yen as change" (10-6-1), which leaves a 6-yen and three 1-yens with P. The next amount to make is Y8, and P pays like 6+1+1 instead of 10-1-1 to best reduce NCC, which leaves no 6-yen and a 1-yen. The next comes Y5, and to minimize the NCC, not 10-1-1-1-1-1 but 10+1-6 is chosen, which leaves one 6-yen and no 1-yen. The next is Y4, but both 6-1-1 and 10-6 leaves the same NCC. In such cases, P favors reducing 6-yens (N-yens) over 1-yens.
Thus, the simulation test was conducted on the 8 C2Ds, with each the same sequence of 100,000 random amounts of Y1 to Y9, and with each starting with P carrying no N-yen and no 1-yen: these conditions are applied the same on the other simulation tests. On test C, NCC[N] is multiplied by 1.61 in calculating QC as test A. table 2-7 shows test C results of all C2Ds.
(3.4) Test D: LC of TPC in CP
Test D is also a computerized simulation, seeking for a minimum LC of TPC. On test D, P chooses every time the paying pattern of a minimum LC. But LC is different from that of test B because of the presence of change and 10-yens. Before conducting test D, I set a new variable DD (Double Digit value) to better reflect the load of count. DD is based on the idea that calculation becomes complicated when the amount affects the higher place. Examples are in the next paragraph. With the introduction of DD, LC is the sum of NCM[1], 1.5 times NCM[N], and DD.
In the case of 1,8C, computerized cash transactions go on like table 2-8. Suppose P carries sometime no 8-yen, one 1-yen, and enough 10-yens. Now, the first amount to make is Y9, and P pay like 10-1, which leaves no 8-yen and two 1-yens with P. In this transaction, DD is 1 because the pay (including 10-yens) exceeds Y9, or reaches 10-yen's place. And LC is 1+1=2. The next amount to make is Y2, and P pays like 1+1 (LC=2) instead of 10-8 (LC=2.5) to choose the smallest LC, which leaves no 6-yen and no 1-yen. DD is 0 if pay is Y9 or lower. The next amount is Y4, and 10+10-8-8 is chosen. This DD is 2 because not only the pay reaches 10-yen's place, but change also exceeds Y9 (excluding 10-yens).
Table 2-9 shows all the C2Ds' results of test D. However, NCC of test D is problematic. For instance, test D of 1,7C leaves P with more than 10,000 1-yen coins at the end of 100,000 transactions. Some other C2Ds also find dozens of NCCs at times. See table 2-10. Such situations are impossible in the real world.
(3.5) Test E: Amended LC of TPC in CP
Therefore, P cannot continue choosing the minimum LC, and has to pay otherwise. I revised the pay patterns of test D to arrange test E. In test E simulation, P usually chooses the smallest LC according to NCC[N] and NCC[1]. But if NCC[1] exceeds 5, P pays the other way to receive the least 1-yen coins as change. When NCC[N] exceeds 5, P switches pay patterns similarly. There can be other ways to keep NCC from overflowing, however, I adopted this method to minimize the increase of LCs. Table 2-11 explains a part of test E simulation in the case of 1,3C.
Test E results are at table 2-12. Although each C2D's LC is somewhat larger than LC of test D (table 2-9), all the NCCs are under control (table 2-13).
(3.6) Tests in higher CP
The above tests A to E were conducted within 1-yen's place, but the results can be applied to other places. In fact, in 10-yen's place, because both amounts to make and the face values of coins used become tenfold, the tests have the same results as those in 1-yen's place. So do the tests in 100-yen's place.
(3.7) Evaluation of C2Ds in CP
To evaluate the 8 C2Ds' test results, I use Ex‰ (Excess Permillage value). This numerical value indicates how inefficient a C2D is, whether QC or LC. A smaller Ex‰ is better, and the best Ex‰ is 0. See table 2-2.
Considering the transaction ratio of TPO to TPC, I integrate all the test results by mixing 5% test A, 5% test B, 45% test C, and 45% test E (table 2-14). The best C2D turned out definitely 1,4C: it marked perfect records on all the tests. It remains the first if the mix ratio of tests A to E is otherwise. 1,6C came in the second place, followed by 1,7C and 1,3C. Meanwhile, 1,5C, still the choice of humankind as of 21st century, ranked 5th to disclose its poor efficiencies.
(4) Tests in banknotes' low places
In BP (Banknotes' Places), I investigate C2Ds for their efficiencies through some tests that are similar to those in CP. QC on coins' tests changes its name into QB (Quantity of Banknotes), and LC is another indicator on tests in BP, too. Transaction types in BP are whether TPO or TPC, and the vast majority would be TPC, the same as in CP. However, there are some differences between hard money and paper money.
(4.1) Decreasing 9 frequencies
In CP, I assumed that the amounts of 1 to 9 made in real cash transactions appear in even frequencies, and did tests A to E without paying attention to this point. Indeed, the frequencies of Y1, Y2, ... Y9 would be nearly equal. Y10 to Y90 in 10-yen's place would follow suit. 100-yen's place might have a gentle decline, though, its effect should be limited and far from overturning the 1,4C's preeminence. Incidentally, the frequency of 0, a meaningless factor in comparing the 8 C2Ds, might somewhat be higher than the others because prices of goods and services are often set at a rough value, or the amount whose HN has a small number of digits.
In BP, the frequencies of 1 to 9 are not even. As prices get higher, cash transactions as well as purchases themselves become less often, resulting in higher frequencies of smaller numbers and lower frequencies of larger numbers. Then, the 9 frequencies are in the shape of D9F (Decreasing 9 Frequencies).
(4.2) Restocked denomination
BP is different from CP not merely by its D9F, but also by the fact that people restock their wallets with notes if necessary, from their bank accounts or the safes in their houses. Depending on the place of restocked denomination, I classify banknotes into two: BLP (Banknotes' Low Places) or BHP (Banknotes' High Places). BLP is the places where banknotes are not restocked with. And BHP is the place where D[N] is restocked with.
For example, British people would restock with 20-pound or 50-pound banknotes, which makes 10-pound's place to be BHP. If you live in one of EU nations and restock with 20-euro or 50-euro, and usually do not carry 100-euro or larger notes, then 10-euro's place falls into BHP. But if you restock with 100-euro, 10-euro's place turns to BLP. Should you restock with 200-euro or 500-euro, then 10-euro's place is BLP and 100-euro's place is BHP. Thus, BLP and BHP could vary from person to person due to differences in their cash spending habits.
The place where D[1] is restocked with is classified as neither BLP nor BHP. If you restock with 100-euro banknotes in the former paragraph, 100-euro's place belongs to neither of them. There is no need to compare the 8 C2Ds in such places because each C2D makes no difference in the number of that sole D[1]. Then, I first study BLP.
(4.3) D9F based on lognormal distribution
The shape of D9F is expected to be lognormal distribution (figure 1), a continuous probability distribution that typically represents the relationship between income and population. I use this function to set D9F for BLP. But what should the decreasing rate be so that D9F well reflects the reality?
Figure 1
Lognormal distribution
¤ f(x)=1/x/√(2πe(log(x))2). μ=0. σ=1.
¤ Mode=1/e=0.368. Median=1. Average=√e=1.649.
Some people pay in cash even for a pricey purchase. While others use credit cards or electronic money for most of their shopping and use real money only for cheap purchases. Therefore, D9F in a certain place varies from person to person. To include such various D9Fs, I arrange gradual D9Fs, medium D9F, and steep D9F based on lognormal distribution. See table 4-1, 4-2, and 4-3. Next, I take the average of the three D9Fs to set a D9F for BLP (table 4-4, figure 2).
Figure 2
Steep, medium, gradual, and average D9F
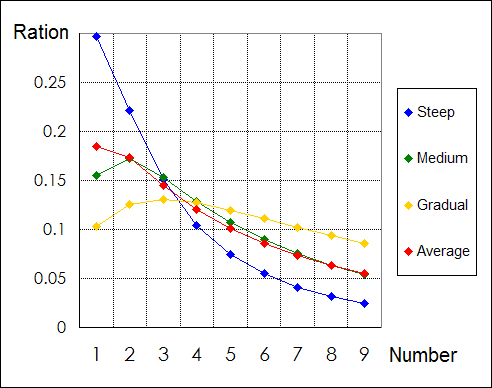
Here I show some practical applications of D9Fs derived from lognormal distribution. For example, in the United States where non-cash payment is common, a woman might have such a cash spending habit as: mode=$4, median=$10, average=$16. Then, gradual D9F just explains 1-dollar's place of her cash transactions. Meanwhile, in Japan where real money still prevails despite being far away from Brazil, a man might usually pay in cash. His data could be: mode=Y900, median=Y2,500, average=Y4,100. Exactly, 1,000-yen's place of his cash use fits steep D9F.
(4.4) Test A2: QB of TPO in BLP
QB depends on NBC (the Number of Banknotes a payer Carries) and each denomination's quantity. The quantity of banknote is determined largely by its area, or length by width: thickness and mass have only a little impact on the quantity of banknotes in daily use. So I searched world banknotes for their areas. See measurements of banknotes. Here, the key is R[N:1], not the area itself. All R[N:1]s concentrated on 1 or a bit larger, though, I took geometric mean in harmony with coins' R[N:1]. They averaged out at 1.06 (table 3-15). Then, QB is defined as NBC[1] (NBC of 1-yen banknotes) plus 1.06*NBC[N].
Test A2 is a test A for BLP. Denominations used on test A2 are imaginary 1-yen banknotes and N-yen banknotes. For example, test A2 of 1,3C counts minimum NBCs to make Y1 to Y9 without change, using 1-yen and 3-yen banknotes, and then calculates QBs. Each QB here is multiplied by D9F before added up to a total QB. Refer to table 4-5. Total QBs of all C2Ds are shown at table 4-6.
(4.5) Test B2: LC of TPO in BLP
Next, I put the 8 C2Ds to test B2. On test B, I counted NCM[N] as 1.5 times the actual number in LC calculation. Here, I also count NBM[N] (the Number of N-yen Banknotes Moved in cash transactions) as 1.5 times. This is because D[1]'s trait of easier counting applies not only to coins but to banknotes as well. Like test A2, each LC is multiplied by D9F before added up to a total LC (table 4-7). Test B2 results are available at table 4-8.
(4.6) Test C2: QB of TPC in BLP
Test C2 is a computerized simulation that examines QB of TPC. However, here on test C2, the frequencies of 1 to 9 are D9F. I arranged random numbers that expectedly distribute in D9F (Table 4-9), and conducted 100,000 transactions like test C. The test results are at table 4-10. Strangely enough, maximum NBCs and average NBCs are quite similar to those NCCs of test C (table 2-7).
(4.7) Test D2 and E2: LC of TPC in BLP
D9F at table 4-9 is also used on test D2 that studies LC of TPC. The test D2 results are shown at table 4-11, but NBCs, particularly 1,8C, inflated as NCCs did on test D (table 2-10, 4-12). Then, I did test E2 that equips the test E's logic to prevent NBC from swelling. Table 4-13 is test E2 results that replaces a defective test D2. NBCs of test E2 are within a reasonable level (table 4-14).
(4.8) Evaluation of C2Ds in BLP
Test results in BLP are at table 4-6 (test A2), 4-8 (test B2), 4-10 (test C2), 4-13 (test E2). The total performances of the 8 C2Ds are evaluated by integrating these Ex‰ values as were done in CP (table 2-14, 4-15). 1,4C ranked top with only losing to 1,3C on test A2. The second came 1,3C that marked good scores throughout the tests in BLP. 1,7C fared well except for tests A2 and B2 to finish third. Meanwhile, well-known 1,5C recorded a dismal 3-digit score.
(5) Tests in banknotes' high places
In BHP, cash transactions are whether TPO or TPC, and the 8 C2Ds need to be tested for their QBs and LCs. These are the same as BLP. However, test C, a simulation test for QB, has a problem: as people manually restock with D[N] in BHP, there needs a new approach to measuring QBs. As for a simulation for LC, it can be done as previous tests.
(5.1) Decreasing 30 frequencies
BHP is the place where D[N] is restocked with. This does not mean that people do not have cash transactions whose amount is larger than BHP. If rare, there can be. But denominations are absent, actually or at least virtually, in the higher places. Therefore, it might be deficient to consider only from Y1 to Y9. With this in mind, I arranged D30F (Decreasing 30 Frequencies) based on lognormal distribution (table 5-1).
Because people usually restock with the largest denomination for each of them, the decreasing rate of each D30F should relatively be stable compared to that of D9F. Therefore, I use only one decreasing rate to set D30F. The slope of D30F is sharper than that of D9F as BHP is higher than BLP.
In practice, D30F could make sense. Suppose there is a European man who usually restocks with 20-euro or 50-euro banknotes. In this case, 10-euro's place is BHP. And if he uses cash in his daily lives like mode=€5, median=€13, average=€21, then his BHP just matches D30F.
(5.2) Test A3: QB of TPO in BHP
For QB of TPO, I arrange test A3, a test A2 with D30F. QB is calculated from minimum NBCs to make Y1 to Y30 without change, in which imaginary 1-yen banknotes and N-yen banknotes are employed. Note that 10-yen notes are absent here. Table 5-2 shows test A3 of 1,4C. NBC[4] (NBC[N]) is counted 1.06 times like test A of BLP. Test A3 results of 1,2C to 1,9C are at table 5-3.
(5.3) Test B3: LC of TPO in BHP
Next comes test B3 that studies LC of TPO in BHP. Like test B2, LC is the sum of NBM[1] and 1.5 times NBM[N]. And each LC to make Y1 to Y30 is multiplied by D30F before added up to a total LC (Table 5-4). The results of the other C2Ds are as shown at table 5-5.
(5.4) Test C3: QB of TPC in BHP
Test C3 is the simulation test to examine QB of TPC in BHP. The amount to make here is Y1 to Y30, which appears in random. I arranged 100,000 random numbers of 1 to 30 that mirror D30F (Table 5-6). Then, I let a computer carry out consecutive 100,000 cash transactions to learn average QBs. On test C3, P carries some 1-yen banknotes and N-yen banknotes. 10-yen notes do not exist.
In the simulated transactions, P restocks with D[N] when the amount on hand gets below the next amount to make. What is important here in BHP is ABR (the Amount of Banknotes Restocked with), not NBR (the Number of Banknotes Restocked with). Then, how much amount should P restock with at once?
In the real world, if people always carry minimum amounts for the next cash transactions, they have to restock their wallets very often. That seems unlikely. Therefore, people would put on some extra amount in restocking. Meanwhile, if there are many people who restock with some D[N] banknotes whose average amount is 10 or more, there would exist a denomination in the higher place. As a result, people are to restock with the higher D[1] instead of D[N], which turns BHP into BLP. Hence, an average ABR in BHP is expected to be around 10 or less.
(5.5) Three tests C3
First, I arrange three tests C3 to address a variety in ABR. On test C3.2, NBR is the least N-yen banknotes to reach, the amount of shortage in the next transaction plus Y2. On the second test C3.4, the plus amount is Y4. The third test C3.6 has Y6 as the margin.
Table 5-7 shows test C3.4 of 1,2C, which describes how 2-yen banknotes are restocked with in the simulation. The results of the three tests are available at table 5-8, 5-9, and 5-10. Average ABRs of tests C3.2 and C3.4 are almost below Y10, and those of test C3.6 are Y10 or so. The three tests seem to well cover the presumed variety in ABR.
Next, I define QB of test C3 as the average QBs of the three tests C3, which would be a good indicator of the real QB in BHP. The 8 C2Ds' performances are calculated at table 5-11.
(5.6) Test D3: LC of TPC in BHP
Test D3 is another simulation test in BHP. The transactions were conducted with random numbers of D30F (table 5-6). On test D3, P carries some 1-yen and N-yen banknotes. There are no 10-yen banknotes. These conditions are the same as test C3. Table 5-12 shows how the simulation proceeds in the case of 1,5C. The test results are at table 5-13.
(5.7) Test E3: Amended LC of TPC in BHP
On test D3, P chooses every time a minimum LC. Following tests D and D2, however, there finds a flaw: NBC[1]s of some C2Ds exceed 10 at times (table 5-14), though not so serious a problem as the previous tests. Then, I improved test D3 to set test E3. For example, the simulation of 1,7C proceeds like table 5-15. Table 5-16 and 5-17 shows the test E3 results, in which all the NBC[1]s are under good control.
(5.8) Evaluation of C2Ds in BHP
In BHP, the proportion of TPO to TPC would be unchanged from BLP or CP. Therefore, I mix Ex‰s of tests A3, B3, C3, and E3 in the ratio of 5:5:45:45 to get the total performances in BHP. See table 5-18. 1,3C managed to finish first in BHP, escaping from 1,4C that was gaining momentum. Other C2Ds lagged far behind them. 1,5C had the third egg on its face value.
(6) The best C2D
Up until here, I have looked into C2D's efficiencies in CP, BLP, and BHP. BLP and BHP results need to be unified to put the findings into practice, because if there were two C2Ds in BP of a currency, people would be highly likely to make more mistakes in handling banknotes.
(6.1) Evaluation of C2Ds in BP
To unify the two results in BP, it is necessary to set the mixing ratio of BLP to BHP. But the ratios are different among currencies and from person to person. So I guessed an average BLP to BHP ratio with the banknotes' data at measurements of banknotes.
I supposed that a half people restock the largest denomination, and the other half restock the second largest one. And I classified the 14 currencies depending on the number of banknote denominations to speculate the ratio of BLP to BHP (table 5-19). It turned out 29 to 14. Then, Ex‰ values of BLP and BHP are mixed in this ratio to get the total results in BP. See table 5-20.
1,4C had a close match with 1,3C to barely win the BP title. The other 3-digit scorers are indifferent. Although the BLP to BHP ratio was based on my speculation, the outline of the standings remains almost unchanged, if the ratio was as low as 1:1 or as high as 4:1.
(6.2) Possible D9F in coins' high places
Before winding up arguments about C2Ds, I just confirm what I mentioned at paragraph (4.1): that 100-yen's place might have a gentle decline in 9 frequencies. Then, I conduct tests A4 and C4, or tests A and C with D9F, assuming a possible decreasing frequencies in coins' high places. Note that tests B and E with D9F have the same results as tests B2 and E2.
Table 2-15 explains test A4 of 1,4C. Test A4 results of 8 C2Ds are at table 2-16. Table 2-17 shows test C4 results, which have a mysterious parallel with test C (table 2-7). These new results are synthesized at table 2-18 to provide C2Ds' performances in CP with possible D9F.
The total performances of all C2Ds at table 2-18 are generally similar to those at table 2-14. But, the Ex‰ scores should be much nearer to the former CP tests, because high CP would have gentle D9F at best, if it has decreasing frequencies at all. In any case, 1,4C kept perfect records, with no fear of losing in CP.
(6.3) A conclusion of C2D
All the above examinations ended up finding 1,4C the best C2D both in CP and in BP. For example, putting together 1,4C in each place, the yen denominations consist of these: 1-yen, 4-yen, 10-yen, 40-yen, 100-yen, 400-yen, 1,000-yen, 4,000-yen, and 10,000-yen. Other currencies follow suit.
(7) 1,3C's possibilities
It turned out that the best C2D is 1,4C. Then, all the C2D currencies in the world should transfer the current 1,5C to 1,4C? Aren't there any other choices? Paying attention to 1,3C's excellent efficiencies in BP, I seek for its possibilities in practical use.
(7.1) 1,3C only in BP
First, it is conceivable to set denominations whose combinations differ between coins and banknotes. That is, 1,4C for coins and 1,3C for banknotes. In the case of the yen, an array of denominations will be: 1-yen, 4-yen, 10-yen, 40-yen, 100-yen, and 400-yen coins; 1,000-yen, 3,000-yen, and 10,000-yen banknotes. As hard money and paper money are materially distinctive, the two D[N]s should not cause mistakes in cash handling. Then, such a choice seems quite possible.
(7.2) Reviewing R[N:1] in CP
On tests in CP, I set R[N:1] as 1.61. But the ratios indeed have a wide range as shown at measurements of coins. What if the ratio is smaller or larger than 1.61? From the viewpoint of material use, a larger ratio is inefficient. Besides, a larger R[N:1] helps people distinguish various coins in their wallets, though, there are other ways to do so.
For instance, Australian 50-cent coin is not a circle but a dodecagon, while the United Kingdom has a unique heptagon 50-pence coin. UK's 1-pound coin is smaller in diameter and thicker than 2-pound coin. And Japanese 5-yen coin, as well as 50-yen, has a hole at its center. These devices provide an easier distinction among coins even if R[N:1] is around 1, or coins' mass and volume are quite similar.
By the way, how about an R[N:1] below 1, or smaller D[N] and larger D[1]? There really are such peculiar denominations: Australian 1-dollar coin is heavier than 2-dollar coin (table 1-1); so is Japanese 10-yen's place (table 1-6). However, most people would feel counterintuitive or something wrong with these cases. And there seem no rational grounds for the anomalies. Therefore, I deny thinking of an R[N:1] lower than 1.
(7.3) Test A5 and test C5 in CP
Assuming the quantity of D[N] coin is equal to that of D[1], I try tests A5 and C5 in CP. R[N:1] is just 1 instead of 1.61, and QC is simply the sum of NCC[1] and NCC[N]. In these tests, NCC[1]s and NCC[N]s are the same as tests A and C (table 2-2 and 2-7). Then, QCs are easy to calculate (table 2-19 and 2-20).
(7.4) Reevaluation in CP
I sum up the results to reevaluate the 8 C2Ds in CP. Tests A and C are replaced by tests A5 and C5 respectively, while tests B and E are unchanged. The total results are as shown at table 2-21. 1,4C achieved perfect records again. 1,3C improved its scores to nearly rival the champion, marking the tops on the new tests.
In the face of new results in which 1,3C proved its high efficiencies in CP, I cannot help but admit some reason to introduce the second best C2D throughout the places. Although 1,4C is definitely the best C2D, 1,3C can be another choice on condition that R[N:1]s approximate 1 in all CPs. To illustrate 1,3C with the yen, it is: 1-yen, 3-yen, 10-yen, 30-yen, 100-yen, 300-yen, 1,000-yen, 3,000-yen, and 10,000-yen.
(8) C3Ds at a glance
At present, in the early years of the 21st century, currencies on the planet are virtually whether 1,5C of C2D or 1,2,5C of C3D. However, the tests run on C2Ds revealed that 1,5C has a far poor efficiency compared to the top 1,4C. Then, how about 1,2,5C? For starters, I just try some tests A and B to take a look at C3Ds' performances. There are 28 participants in C3D division.
(8.1) Test A6: QC of TPO in CP of C3D
Test A in CP of C3D is test A6. Before starting the test, I have to determine R[N1:1] and R[N2:1]. Here, N2 is larger than N1. Table 1-15 shows R[N1:1]s and R[N2:1]s of C3D coins on the earth. As a result of calculating geometric means, R[N1:1] was set as 1.45, and R[N2:1] was 1.60. Then, QC is the sum of NCC[1], 1.45*NCC[N1], and 1.60*NCC[N2].
Table 6-1 shows test A6 of 1,3,4C. The results of the other 27 C3Ds are at table 6-2. The gold medal went to 1,4,6C. And seven losers' Ex‰ came within 50. A notable 1,2,5C was struggling at 17th place.
(8.2) Test B6: LC of TPO in CP of C3D
Test B6 looks into LC of TPO. For example, test B6 of 1,4,5C is shown at table 6-3. Here, both NCM[4] and NCM[5] are multiplied by 1.5 in calculating LC. Total LCs of 28 C3Ds are available at table 6-4. Top position was shared by five competitors. Five others also tied at Ex‰=24 to form the second group. Meanwhile, 1,2,5C revealed its inefficiency, marking 3-digit score again.
(8.3) Test A7: QB of TPO in BLP of C3D
Next, I move to BLP and arrange test A7 for QB of TPO. Banknotes' R[N1:1] and R[N2:1] are also determined based on measurements of real C3D currencies. Table 3-15 calculated that the average R[N1:1] was 1.05, and R[N2:1] 1.12. Then, QB is defined as NBC[1] plus 1.05*NBC[N1] plus 1.12*NBC[N2].
In BLP, the frequencies of 1 to 9 are D9F, whether C2D or C3D. Then, D9F at table 4-4 is also used on test A7. For example, QB calculation of 1,3,6C is detailed at table 6-5. According to table 6-6 that shows all the C3Ds' QB performances, 1,3,4C achieved a sole victory. Amazingly enough, for billions of disappointed 1,2,5C users, it came in second with Ex‰ 35.
(8.4) Test B7: LC of TPO in BLP of C3D
Fourth test for C3D is test B7 that studies LC of TPO. Like test B6, both NBM[N1] and NBM[N2] are multiplied by 1.5. And D9F is applied in LC calculation, much the same as test A7. Table 6-7 shows test B7 of 1,2,5C. Total LCs of all 28 C3Ds are at table 6-8. 1,3,4C barely kept the sole lead to win the race. 1,3,5C and 1,3,6C were just behind the front runner. 1,2,5C failed to catch up with them and returned to a suitable middle class.
(8.5) Promising candidates of C3D
Here, I stop to review the results of four C3D tests. Although tests done so far are only for TPO (table 6-9), the results have some clues to the best C3D. In CP, 1,4,6C marked perfect records on tests A6 and B6. Then, it is the likeliest candidate. Some other rivals including 1,3,6C and 1,4,5C are well positioned to possibly turn the tables on TPC tests. Meanwhile in BLP, 1,3,4C seems the best C3D, securing some advantages over the others.
(9) C2D or C3D
It seems that the best C3D differs between CP and BP. However, suppose the remaining tests conclude that the overall winner is 1,4,6C in CP and 1,3,4C in BP. Then, which is more efficient, 1,4C of C2D or the best pair of C3D? The question is apparently hard to answer. But here is a cue: as the current denominational combinations, namely 1,5C and 1,2,5C, are competing each other globally, their efficiency levels are expected to be close enough. Based on the assumption, it would be determined by how much each top combination outperforms the current one.
According to table 2-14, Ex‰ difference between 1,4C and 1,5C in CP tests is 79. And that in BP is 103 (table 5-20). Meanwhile, 1,2,5C sees Ex‰ lags by 129 (table 6-2), 119 (table 6-4), 35 (table 6-6), and 90 (table 6-8). Judging from the numerical values available here, both C2D and C3D have improvements by about the same degree. Therefore, I cannot determine which is better.
(9.1) A decisive factor
Further investigation into C3Ds would unravel the true picture. However, without such a seemingly tremendous work, I was convinced by another logic to reach a conclusion. As I mentioned at paragraph (2), C2D has a problem in the number of coins and banknotes. On the other hand, C3D is bothered by a wide variety of denominations. Here, 1,4C succeeded in minimizing C2D's weakness. But the best C3D had no improvement in its inherent defect even if it fortified the original strength. What C3D should do to compete with C2D is to remove its bottleneck, or slash the number of denominations, hence turn into C2D. Therefore, all the combinations, whether C2D or C3D, end up 1,4C.
(9.2) Adhering to C3D
If a C3D monetary system is reluctant for some reason to change into C2D, it may adhere to C3D. However, I have no intention to seek further for the best denominational combination for such C3D adherents. I only recommend them to abandon the wasteful convention and try 1,3,4C, 1,4,6C or something new that sounds harmonious. Taking 1,4C must be a major avenue, though, there are some better C3D ways than the status quo.
(9.3) Premises
All the arguments in this paper make sense for the terrestrial humans as of 21st century. However, there exist 2 premises: first, the number is represented by the decimal system; second, denominations have two modes, or hard money and paper money. These foundations seem to be stable for the time being. Should anything change, some C3Ds might overtake 1,4C.
For instance, suppose the hexadecimal system was introduced in human society. Then, C2D would want another denomination to cover the amounts of 1 to 15 in a place. On the other hand, if the money of third mode, something made of neither metal nor paper, emerged on the earth, coins and banknotes should surrender some denominations to the newcomer. As a result, people would feel less awkward to handle a wide variety of C3D denominations. In the event of such upheavals in future, my study will need to be reviewed.
(10) Conclusions
Lastly, I list here some important conclusions my study has reached.
(1) It is 1,4C that is the best denominational combination whether in CP or in BP.
(2) In BP, 1,3C can be an alternative.
(3) 1,3C can be another choice in CP as well as in BP on condition that all R[N:1]s in CP approximate 1.
(4) Both 1,5C and 1,2,5C are so poor in efficiency as to have gone extinct from the planet.
Abbreviations
1,5C: 1,5 Combination, or the combination of D[1] and D[5]ABR: the Amount of Banknotes Restocked with
BHP: Banknotes' High Places, where D[N]s are restocked with
BLP: Banknotes' Low Places, where banknotes are not restocked with
BP: Banknotes' Places
C2D: Combination of 2 Denominations per place
C3D: Combination of 3 Denominations per place
CP: Coins' Places
D[25]: Denominations of HN=25
D30F: Decreasing 30 Frequencies, which are used in BHP
D9F: Decreasing 9 Frequencies, which are used in BLP
DD: Double Digit value; A variable used in simulation tests for LC; DD is 0, 1, or 2.
Ex‰: Excess Permillage value, which represents inefficiencies of C2D or C3D; A smaller Ex‰ is better, and the best Ex‰ is 0.
HN: Head Number; The number without consecutive 0s from the one's place of a whole number
LC: Load of Count in cash transactions
NBC: the Number of Banknotes a payer Carries
NBC[1]: NBC of 1-yen banknotes
NBM[N]: the Number of N-yen Banknotes Moved in cash transactions
NBR: the Number of Banknotes Restocked with
NCC: the Number of Coins a payer Carries
NCC[1]: NCC of 1-yen coins
NCM: the Number of Coins Moved in cash transactions
NCM[1]: NCM of 1-yen coins
P: a Payer in cash transactions
QB: Quantity of Banknotes, which is determined by NBC and R[N:1]
QC: Quantity of Coins, which is determined by NCC and R[N:1]
R[N:1]: the quantity Ratio of D[N] to D[1] in the same place
TPC: Transaction of Payment with Change allowed
TPO: Transaction of Payment Only, with change not allowed
Y732: the amount of 732 yen
1¤4
An Efficient Combination of the Denominations of a CurrencyTables 1: Measurements of coins
Tables 2: Tests in coins' places
Tables 3: Measurements of banknotes
Tables 4: Tests in banknotes' low places
Tables 5: Tests in banknotes' high places
Tables 6: Tests of C3Ds